The theorem was discovered in the papers of Thomas Bayes, an English Presbyterian minister, and mathematician, and was published posthumously in the year 1763. Bayesian inference, also known as Bayesianism, is related to the theorem because it is based on the assignment of some a priori distribution of a parameter under investigation. In probability theory, Bayes’ theorem is a method for revising predictions in light of new evidence. The other name for Bayes theorem is conditional probability or inverse probability.
Definition of Probability
Probability is a branch of mathematics that deals with numerical descriptions of how likely an event is to occur or how likely a proposition is to be true. The probability of an event is a number between 0 and 1, where 0 indicates the event’s impossibility and 1 indicates certainty.The greater an event’s probability, the more likely it will occur. Tossing a fair (unbiased) coin is a simple example. Because the coin is fair, the two outcomes (“heads” and “tails”) are both equally likely; the probability of “heads” equals the probability of “tails,” and because no other outcomes are possible, the probability of either “heads” or “tails” is equal to 1/2. (we can also write it as 0.5 or 50 percent ).
Let’s discuss probability and Bayes theorem in probability.
Bayes Theorem in Probability
The Bayes theorem is a probability as well as statistics theorem named after Reverend Thomas Bayes that aids in determining the probability of an event based on a previous event. The Bayes theorem has many applications, including Bayesian inference, determining the likelihood of developing health problems as one gets older, and many others. With the help of examples, we will attempt to understand the use of the Bayes theorem in determining the probability of events, its statement, formula, and derivation.
Formula For Bayes Theorem
In layman’s terms, the Bayes theorem determines the conditional probability of an event A given that event B has already occurred. The Bayes theorem is also referred to as the Bayes Rule or the Bayes Law. It is a method for calculating the likelihood of an event based on the occurrences of previous events. It is employed in the computation of conditional probability.
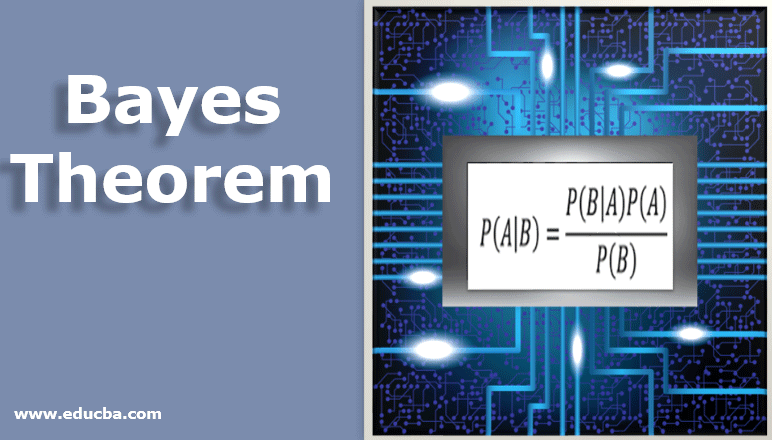
The Bayes theorem computes probability based on a hypothesis. Let us now state the theorem and its proof. According to the Bayes theorem, the conditional probability of an event A given the occurrence of another event B is equal to the product of B’s likelihood given A and A’s probability. It is written as follows:
| P(A|B)=P(B|A)P(A)/P(B) |
In this case, P(A) denotes how likely A is to occur (Prior knowledge)- the probability that a hypothesis is correct before any evidence is present.
P(B) in the formula denotes the likelihood that B will occur.
(Marginalization)- The likelihood of seeing the evidence.
P(A/B) in the formula denotes the probability that event A will occur given that event B has occurred.
(Posterior)
The likelihood that a hypothesis is correct given the evidence.
P(B/A) denotes the likelihood that event B will occur given that event A has occurred (Likelihood)- the likelihood of seeing the evidence if the hypothesis is correct.
Example For Bayes Theorem
If a patient is an alcoholic, you might be curious about their chances of developing liver disease. “Being an alcoholic” is the litmus test for liver disease.
- A could denote the occurrence “Patient has liver disease.” According to previous data, 10% of patients who enter your clinic have liver disease. P(A) equals 0.10.
- B could stand for the litmus test, “Patient is an alcoholic.” Alcoholism affects 5% of the clinic’s patients. P(B) equals 0.05.
- You may also be aware that 7% of those diagnosed with liver disease are alcoholics. This is your B|A: a patient with liver disease has a 7 percent chance of being an alcoholic. If you want to understand the topic in a fun and interesting manner you may visit Cuemath.